Chapter 13, Problem # 7
a) When a star begins to move off the main sequence, it has used up all the
hydrogen in its core, thus fusion has ceased there. Without the pressure
produced by heating due to fusion, the core collapses. As the core contracts,
it releases gravitational energy which creates heat and pressure which
pushes on the outer layers of the star, causing them to expand, and so the
star becomes a giant.
Thus, although the core of the star does collapse under the force of gravity,
the gravitational energy released by its contraction causes the outer layers
of the star to expand rather than contract, and the star initially becomes a
giant.
Gravity ultimately wins out because the collapsing core does not ever get hot
enough to ignite any further nuclear reactions. Thus the collapsed core (which
is initially heated by the release of gravitational energy) will ultimately
cool off. Then there will be no more pressure to counteract the force of
gravity, so gravity will win and the star will contract.
b) There are many red giants visible in the sky even though they are relatively
short-lived (and thus relatively rare among the population of stars), because
red giants are very luminous. Thus, all the red giants out to a great distance
from the earth, not just those relatively nearby, are visible in our sky.
Chapter 13, Problem # 9
a) Stars with masses from 0.1 solar masses to a few solar masses will
eventually become white dwarfs.
b) Stars with masses from a few solar masses to about 10 solar masses will
become neutron stars.
c) Stars with masses greater than about 10 solar masses will eventually become
black holes.
The Sun will eventually become a white dwarf.
Chapter 13, Problem # 10
A main sequence B3 star has a mass of approximately 10 solar masses.
The relationship between mass and luminosity on the main sequence is
L = M3.5 (in solar units).
So a star with 10 times the mass of the sun has a luminosity of
103.5 = about 3000 times that of the Sun.
The estimated lifetime of the star is the quantity of fuel it contains (M),
divided by the rate at which it consumes that fuel (L):
M/L = 10/3000 = 1/300 times the lifetime of the Sun.
Finally, we note that more massive stars burn a larger percentage of
their hydrogen supply than do less massive stars. So the actual lifetime
of the star is probably a bit longer than the number calculated above,
giving a lifetime for the B3 star of around 1/200 times the lifetime of
the Sun.
Chapter 14, Problem # 8
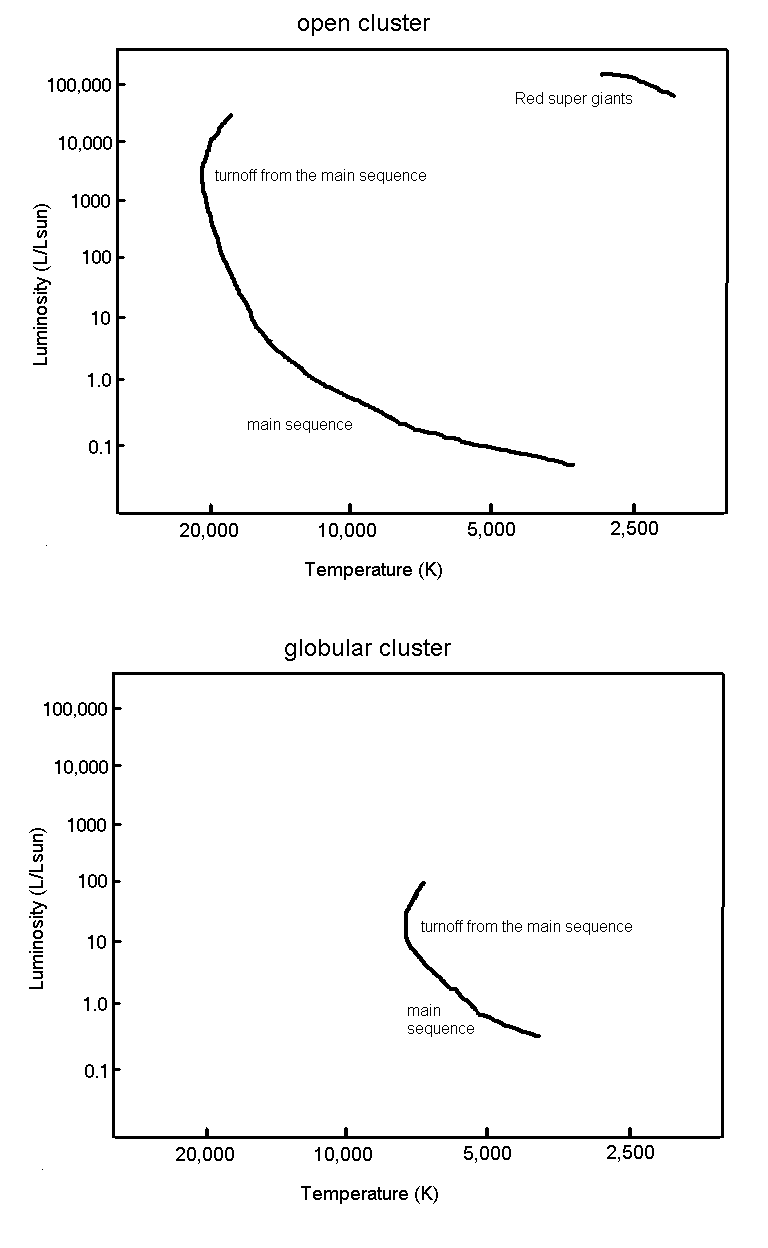
Below are sample plots of an open cluster and a globular cluster. Your plot
may vary somewhat from these. Since the open cluster is far younger than
the globular cluster, it contains a longer main sequence stretching out to
the very luminous stars which have short lifetimes. The open cluster
plot may also contain red supergiants. The globular cluster contains
only the longer-lived less luminous stars in the main sequence, and it may
contain red giant stars as well (although the sample shown below does not).
Chapter 14, Problem # 9
The difference in apparent brightness between the H-R diagrams of two
clusters is related to the relative distances between the clusters
according to the inverse square law:
ratio of apparent brightness = 1/(ratio of distances)2
or equivalently
ratio of distances = 1/(ratio of apparent brightnesses)1/2
a) So if cluster one is 35 times fainter than cluster two, then:
(distance2)/(distance1) = 1/(35)1/2
distance1 = (35)1/2 × distance2
Given that the second cluster is at a distance of 120 pc, we find:
distance1 = (35)1/2 × 120 pc =
710 pc
b) If cluster one is 76 times fainter than cluster two, then
(distance2/(distance1) = 1/(76)1/2
Again using that the distance to the second cluster is 120 pc, we get
distance1 = (76)1/2 × 120 pc =
1046 pc
