Third peak detected in Microwave Backround?

In May 2001, the experiments DASI, BOOMERanG, and Maxima-1 all reported new (or extended) measurements of the angular power spectrum of the cosmic microwave background. All data sets appear to show both second and third peaks. Many in the cosmological community gave a sigh of relief, as the third peak appears too large to be consistent with the previously successful no-CDM prediction I had made:
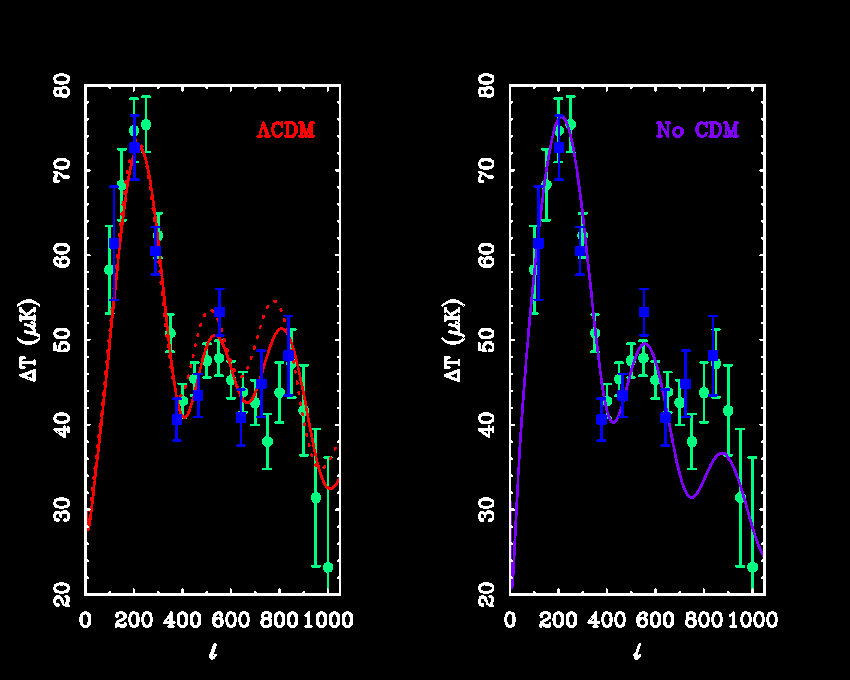The new data from DASI (blue) and BOOMERanG (green) together with LCDM (left panel) and no CDM (right panel) models. [The Maxima-1 data have been excluded because the addition of more points and error bars overwhelms the eye.] The solid red line is the best constrained fit of the BOOMERanG preprint (from the last line of their Table 4). The dotted red line is the pre-existing LCDM model (Turner 1999) which comes closest to the data. The purple line is the pre-existing no-CDM model of McGaugh (1999), unmodified from the fit to the [old, first release] combined BOOMERanG and Maxima-1 data (which, except for a very slight tweak to the geometry, is identical to the no-CDM models discussed by McGaugh 2000).

At first glance, it does appear that the there is more power in the third peak than predicted by the no-CDM model. This is, as emphasized by McGaugh (1999), the simplest expression of a possible MOND model. Reality could be, and at some level must be, more complicated. However, it is not yet necessary to invoke this to "save" the no-CDM model, which actually still does quite well:

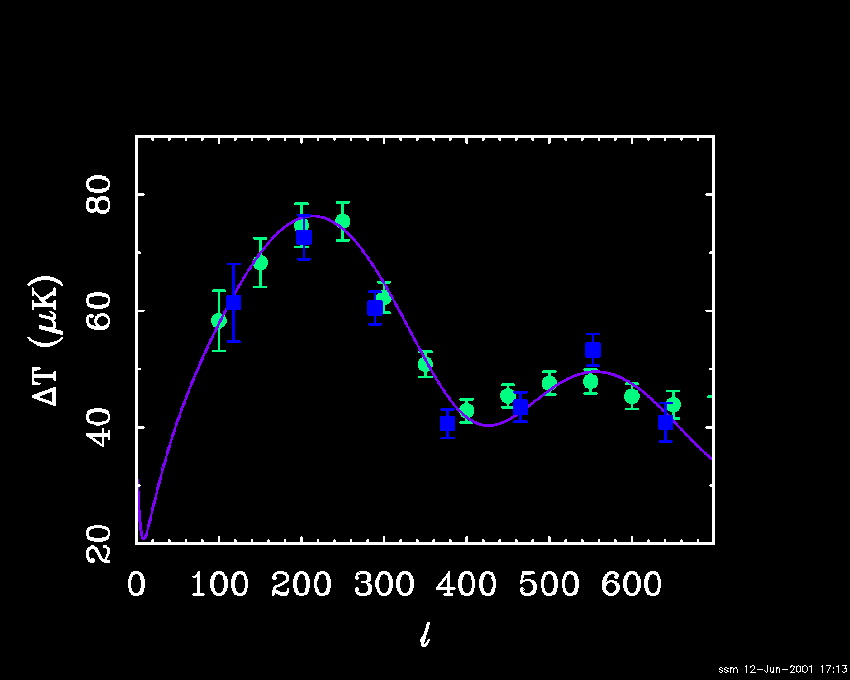A close up of the first two peaks detected by BOOMERanG and DASI, together with the pre-existing no-CDM model. The model has not been adjusted in any way from what was previously published. [Not even the amplitude has been adjusted: the BOOMERanG recalibration agrees eerily well with the old fit to the MAXIMA-1 normalization.]

Goodness of fit with no attempt to re-fit model and accounting only for tabulated errors. Systematic errors start to dominate at large l, and completely dominate l > 700.

Experimentchi^2chi^2 (L<700)
BOOMERanG1.830.76
Maxima-11.9*1.63
DASI2.981.94+
Combined2.11.34&
*chi^2 budget dominated by point at L=147. Without it, chi^2=1.25
+chi^2 budget dominated by point at L=289. Without it, chi^2=1.19
&chi^2=0.85 excluding above 2 points (remaining N=26)

Note that the chi^2 budget is dominated by points with small error bars at low l. Any model which fits the first peak as defined by the remainder of those points will pay a comparable penalty.

The only "problem" is with the putative third peak, where the uncertainties are still quite large. In addition to the random errors in the above figures, there is also a systematic error due to the uncertainty in the BOOMERanG beam size. This dominates at large l, and can cause a tilt of the spectrum:

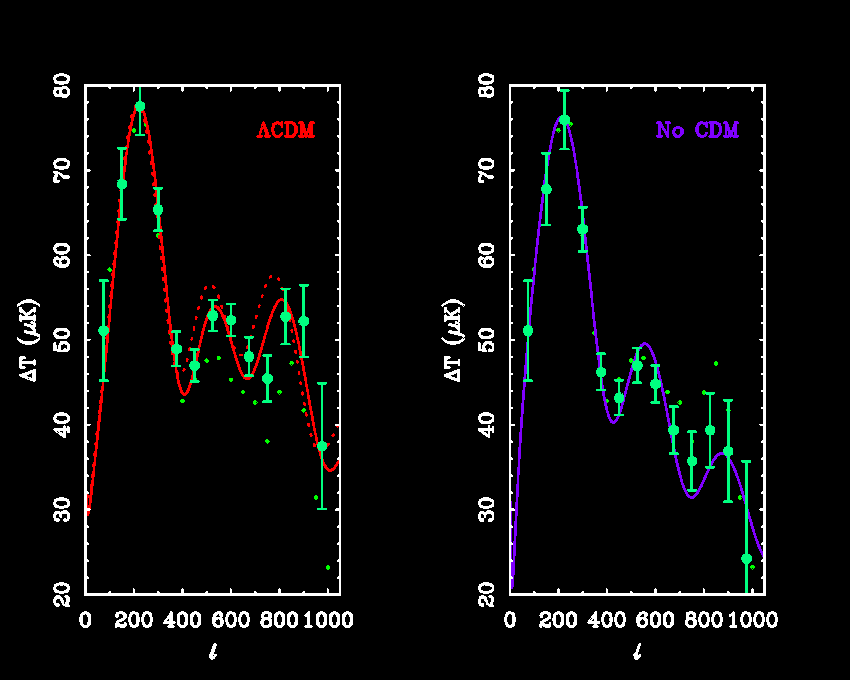The BOOMERanG data with the tilt resulting from the effects of a +/- 1 sigma error in the beam size (the little triangles in their Fig. 2). The data have been tilted by 1 sigma in the direction favorable to CDM in the left panel and by 1 sigma in the opposite direction in the right panel. The untilted data are shown by small green dots. As a result of this beam uncertainty, the data are consistent with either LCDM or no-CDM models. The model lines are identical to those in the figure above except that the normalization of the red lines has been adjusted to better match the tilted data.

As a result of the systematic beam uncertainty, the simple no-CDM model remains quite viable. Indeed, it is only because of this uncertainty that any model provides a decent fit. Without it, the chi^2 of the "best" fit LCDM model is quite horrid.

So, where are we? Unfortunately, I don't think the new data shed any additional light on the issue of the existence of CDM. It still appears that the second peak is smaller than had been expected by LCDM models, roughly consistent with no-CDM expectations. But even this may now be in doubt, as the beam uncertainty does begin to play a role even here. The amplitude of the third peak remains too poorly constrained to be of any use.

So, what'll it take? In principle, a good measurement of the first three peaks ought to do it. Ideally, this should come from the same experiment so as to minimize issues of calibration. MAP should certainly nail the first two peaks, which will help a lot. It is not clear how much it will help with the third. Perhaps enough by itself; or perhaps in combination with other experiments.

So, what will happen?
 Some further predictions of the no-CDM model:

These are all expectations within the framework of a purely baryonic, CDM-free universe. This, in turn, is only the first-order approximation of a MOND prediction. Ultimately, MOND must require some deviation from this. For example, if MOND is a modifcation of intertia, the effective mass of the baryons may be less at low accelerations, resulting in less baryonic drag. If instead MOND is a modification of gravity, then the integrated Sachs-Wolfe effect is probably underestimated and the pure baryon model which fits the peaks will underpredict the COBE data. Maybe there will be a clear signature of physics beyond the existence or nonexistence of CDM in the MAP data, but that in itself should be a wonderful thing!

© Stacy McGaugh, May 8, 2001; last edited July 18, 2001

Return to the MOND pages home page


Lawrence Pretended Not to Notice That a Bear Had Become Attatched to His Coattail
from A Journey of the Imagination © James Christensen