
The External Field Effect
One of the curious aspects of MOND is the External Field Efect (EFE).
Because the theory violates the strong equivalence principle
(but not necessarily the Einstein or weak equivalence principle),
one can not ignore the influence of external
masses that impose an acceleration gext on systems with
small inernal accelerations gin.
[The EFE often seems to get confused with a putative Internal
Field Effect, in which high internal accelerations of a sub-system
(like a solar system) are imagined to preclude that sub-system from obeying MOND
within a larger system (like a galaxy). This is a misconception. The
external field of the galaxy might affect what goes on in a sub-system
like a solar system; the internal motions of the sub-system are irrelavant
to how it moves in the larger system. Only the center of mass of the
sub-system matters to that (see below).]
There are four broad regimes, each with an applicable mass estimator:
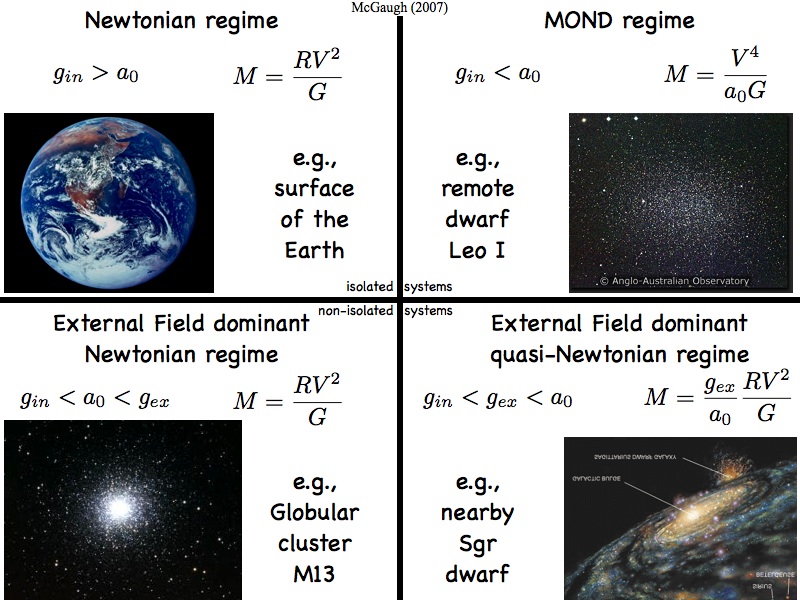
Note that for this illustration, the mass estimator assumes that one is
comfortably in an extremal regime (g << or >> a0).
There can be small corrections due to the interpolation function
μ(g/a0) when g is within a factor of a few of a0.
In words, these regimes are:
- Newtonian Regime: gin > a0
A system with accelerations well in excess of a0
behaves in a purely Newtonian manner: g = gN.
(For a point mass M, gN = GM/r2.)
Examples of systems where this occurs:
- The Earth
- The solar system
- The inner regions of elliptical galaxies
- MOND Regime:
gext < gin < a0
A system with accelerations well below a0
but above those imposed by any external source
is in the MOND regime: g = √(gN a0).
Examples of systems where this occurs:
- Low Surface Brightness Galaxies
Any diffuse, isolated extragalactic system with surface density
< a0/G (≈ 860 M⊙ pc-2
≈ a sheet of paper).
- The outer regions of high surface brightness galaxies
- Externally Imposed Newtonian Regime:
gin < a0 < gext
In this case, we imagine a system that has very small internal accelerations
but which is influenced by a larger system that imposes accelerations in the
Newtonian regime. In this case, the large external acceleration "wins" and
the internal behavior is purely Newtonian irrespective of how small the
system's internal accelerations may be.
Examples of systems where this occurs:
- Eotvos type experiments
Experimentalists have devised systems with internal accelerations several
orders of magnitude smaller than a0. These do not detect
MONDian effects, nor should they. The experiments sit on the surface of
the earth, where gext = 1011a0, so the
internal dynamics behave in a purely Newtonian fashion.
- Star clusters or binary stars in the solar neighborhood
For current (2011) estimates of the Galactic constants, the solar
neighborhood has a centripetal acceleration about the Galactic center of
approximately 1.8a0. Therefore binary stars and local star
clusters are still marginally in the Newtonian regime and should not
exhibit pronounced MONDian behavior, even when their internal accelerations
fall below a0. (I say "pronounced" because 1.8a0
is close enough to the MOND regime that there can be small deviations
from purely Newtonian behavior as the interpolation function
μ(x = g/a0) starts to deviate from the Newtonian limit
(μ(x) → 1), which strictly applies only for x >> 1 [i.e.,
g >> a0].)
- Galaxies in the cores of rich clusters of galaxies
Some very large clusters obtain accelerations close to or even in excess
of a0 in their central regions. This might cause morphological
transformations as spirals that venture through this region lose the
stability imparted by MOND.
NGC 4438
comes to mind. Exactly what happens would depend on
how long the spiral was subject to how large an external acceleration,
but this might go some way to explain why ellipticals dominate the cores
of rich clusters.
Note that this is where the violation of the strong equivalence principle
is most obvious - a system of interacting particles cares about the
external universe in a disturbingly Machian sort of way.
- Quasi-Newtonian Regime:
gin < gext < a0
A system in which all accelerations are in the MOND regime but where the
external acceleration exceeds the interal acceleration is in the
Quasi-Newtonian Regime. Here the behavior is Newtonian in the sense
that the effective force still follows the inverse square law, but the
effective value of G is enhanced by the factor a0/gext.
(Strictly speaking, by the inverse of the interpolation function
1/μ(x) where x = gext/a0.)
Systems in this regime will show a mass discrepancy (M will be interpreted
to be enhanced instead of G) but may also show a Keplerian decline in their
rotation curve. Ultimately, all extragalactic systems must reach this
regime at some point, as the acceleration due to the rest of the universe
exceeds that of the central object. So rotation curves should not stay
flat forever, even in MOND, just because there are other masses in the
universe.
Examples of systems where this occurs:
- Dwarf Spheroidals
Many of the dwarf satellites of the Milky Way and Andromeda are in the regime
where the external field of the giant host dominates over the internal field
of the dwarf. A test particles in orbit around such dwarfs at many optical
radii (e.g., tidal debris) would show a Keplerian fall-off around a central
mass inferred to have lots of dark matter.
This is a cautionary example where it would be very easy to wrongly
interpret such an observation as falsifying MOND because V(r) does not
remain flat and the edge of dark matter subhalo appears to be encompassed.
- The Milky Way
Andromeda is the next large system in the Local Group, and is a bit
more massive than the Milky Way. It should take over around 300 kpc,
so we would infer an "edge" to the dark matter halo of that order
(the exact radius depending on how we chose to define the "edge").
In general, all galaxies would be inferred to have an edge to their
phantom dark matter halos simply
because there comes a point when the rest of the universe takes over.
In the case of the Milky Way, this extent would be directionally dependent
due to the presence of Andromeda.
- Lyα clouds
These insubstantial wisps of gas are usually so diffuse that their internal
accelerations are less than the external acceleration imposed by the
neighboring large scale structure.
See Milgrom's explanation for more on
why this happens in MOND. See also the
MOND laws of galactic dynamics.
Not the Internal Field Effect
The External Field Effect is often confused with what I'll call the
Internal Field Effect (IFE) to distinguish it. The EFE is the effect
of the field of a very large system on a much smaller sub-system. The putative
IFE is the effect of the accelerations within a sub-system on how that
sub-system behaves within the larger system.
An obvious example is a solar system orbiting within a galaxy.
The solar system has Newtonian accelerations, so isn't it in the Newtonian
regime, and therefore immune to MONDian effects? The answer is no -
the internal accelerations of the sub-system are irrelevant to how that
sub-system responds to an external field. Only the field of the parent system
at the position of the center of mass of the sub-sytem is relevant to that.
Much as we do not need to know the quantum mechanical motion of all the
particles that compose a baseball to understand the macrosopic motion of its
center of mass, so too is the internal structure (and accelerations) of a solar
system irrelevant to how the center of mass of the system orbits in a galaxy.
A Newtonian solar system is a billiard ball to a MONDian galaxy.
There is a boundary condition that defines the edge of the billiard ball,
roughly where gin ~ gex. This occurs at about 7,000 AU
for our solar system. Everything inside of that boundary is part of the
internal structure of the system. While the EFE can reach inside that boundary,
the IFE cannot reach outside it. Only the center of mass of such systems
matters to determining their orbits in MOND, not their internal
structure, nor the magnitude of their internal accelerations.
That is to say, MOND respects the weak equivalence principle:
the motion of a particle - be it a billiard ball or a solar system -
is independent of its internal structure or composition.
This page
in Polish.
This page in Russian.


