
Frequently Asked Questions
Q: What is MOND?
A: MOND stands for MOdified Newtonian Dynamics. It is a modification
of the usual Newtonian force law hypothesized in 1983 by Moti Milgrom
of the Weizmann Institute
as an alternative to Dark Matter.
Q: What is the modification?
A: MOND can be interpreted as either a modification of
gravity through a change to the Poisson equation, or as a
modification of inertia through a breaking
of the equivalence of inertial and gravitational mass.
The modification occurs at very small accelerations. Above a critical
acceleration a0 (the one parameter of the theory), everything
is normal. Below a0, the effective acceleration approaches
a = (gN a0)1/2, where gN is the
normal Newtonian acceleration. The two regimes are joined smoothly by
an interpolation function mu(x) with the asymptotic property mu(x) → 1
for x » 1 and mu(x) → x for x « 1, where x = a/a0.
In the case of a modification of the Law of Inertia, a=F/m
becomes a=F/[m*mu(x)], and the effective acceleration can be found from
a*mu(x) = gN.
In the case of a modification of the Law of Gravity, the Poisson equation
becomes
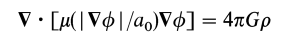 ,
which is well approximated by a*mu(x) = gN.
,
which is well approximated by a*mu(x) = gN.
Q: Isn't it rather drastic to modify such fundamental Laws?
A: Sure. Just as it is drastic to fill the universe with non-baryonic cold
dark matter consisting of new fundamental particles we don't actually know
to exist. The data drive us to one of these extremes. The question, of
course, is which is a better approximation of Nature?
Q: What is mu(x)?
A: The interpolation function is not specified theoretically, but there is a
fairly narrow range of empirically acceptable mu(x). Possible forms
which are acceptable to galaxy data are
mu(x) = x(1+x2)-1/2
mu(x) = x/(1+x), and
mu(x) = 1-e-x.
Q: What is the acceleration scale?
A: a0 = 1.2 x 10-10 m s-2, i.e.,
about one Angstrom per second per second. This is one part in 1011
of what we feel on the surface of the earth. The precise value depends on
the distance scale to galaxies, so perhaps it would be better to say
a0 = 1.2 x 10-10 m s-2
h752, where h = H0/75 is the
Hubble Constant (the expansion rate of the universe) in units of
H0 = 75 km s-1 Mpc-1.
(Currently, most measurements report values in the neighborhood of
H0 = 72 km s-1 Mpc-1.)
Q: Why an acceleration based modification?
A: We usually think first in terms of a modification at some length scale:
galaxies are big, so maybe gravity is different on large scales. This does
not work. But there are other scales which are different about galaxies.
One of them is the very low centripetal acceleration experienced by stars
orbiting within galaxies. This is just as far removed from our laboratories
as is the size scale of galaxies.
MOND was motivated by two observations: 1) the asymptotic flatness of rotation
curves and 2) the slope of the Tully-Fisher relation
(M ~ Vflat4). These two things lead to an acceleration
scale:
Newton: GM/R = V2
Observed: M = AV4
where A is a constant which holds
irrespective of differences in R. Squaring
Newton,
V4 = (GM/R)2 = M/A
A-1 = G2M/R2 = G*(G*surface density)
and G*surface density has units of acceleration:
A-1 = Ga0
Q: MOND fits the rotation curves of spiral galaxies well, but it was designed
to do that. So do such data provide any test?
A: Yes.
That MOND was "designed" to fit rotation curves and is therefore guaranteed
to do so is a common misconception.
Both MOND and dark matter were invented
to explain the presence of mass discrepancies in astronomical data,
especially the flat rotation curves of spiral galaxies. It is often
asserted that MOND is ad hoc (and therefore bad), but this is also
true of dark matter. Once invoked, dark matter can be distributed
in any way necessary to explain just about anything. It is difficult to
test, and does not constitute a falsifiable theory. In contrast,
once a modified force law is specified, there is no freedom to adjust
its predictions.
MOND can not fit any arbitrary rotation curve:
it is tied down by the observed mass distribution.
However, it does fit real data. I find it remarkable that of
the infinite variety of things rotation curves
might plausibly do were they caused by a Newtonian disk + dark matter halo,
they in fact do the one (and only one) thing allowed by MOND.
Fundamental Clue or Big Cosmic Accident? (Is God subtle or malicious?)
MOND's detractor's often assert it is just some
sort of fluke, so I guess they prefer to believe that God is malicious.
Q: But wait, there is a fundamental objection to an acceleration scale.
The atoms within stars have acceleratoins in the Newtonian regime.
Since the star's constituents are Newtonian with a > a0,
the star itself can never be in the MOND regime.
A: This comes down to when you can consider an object to be a
billiard ball, as
we are so fond of doing in physics. Is a star an unremitingly Newtonian
object because of the microscopic motion of its constiuent atoms? Or can
it be treated as a point mass in the much larger gravitational field of
the entire galaxy?
It would be quite a thing if the accelerations of atoms within stars
could affect their motion in galaxies. There is a matching condition
between the Newtonian and MOND regimes. This effectively defines the
location at which you are in one regime or the other - and whether things
interior to that regime can be treated as a single billiard ball for the
purpose of calculating its motion, e.g the barycenter of the solar system
about the galaxy. The solar system is Newtonian out to about a tenth of a
light year; all the substructure we are familiar with is well within this
radius and matters not at all to the galactic motion.
If quantum mechanics has taught us anything, it is that there is
a regime where substructure matters, and another where the billiard-ball
approximation is quite good. Whether this analogy extends to MOND or not
I expect will be debated for many years to come. It seems not
unreasonable that it might.
Q: Wouldn't MOND effects show up in precision solar system tests?
A: They may have already. Whether they
should or not depends on the interpolation function mu(x).
The accelerations in the inner solar system are millions of times higher
than the MOND acceleration scale, so all that can be tested here is the
(slight) deviation of the interpolation function from unity. This might
be perceptible if mu(x) approaches unity slowly in the limit x » 1, but
would not be if it converged rapidly. For example, if mu(x) = 1-e-x,
then the deviation one is looking for is of order one part in
e108.
MOND and General Relativity
Q: Does MOND contradict General Relativity?
A: It generally seems to be assumed that it must, but this is not true.
GR and MOND apply in very different regimes. Relativity is what happens
as v → c; MOND is what happens as a → 0.
Empirically, these regimes couldn't be further removed.
Q: Is there a complete relativistic theory incorporating MOND?
A: There have been suggestions, such as Bekenstein's
Phased Coupled Gravity and Sanders's
Stratified Scalar-Tensor Theory.
It is not obvious that either of these are satisfactory, but they do
at least demonstrate that it is possible to construct theories which
encompass both GR and MOND.
This is a hard problem, especially conceptually. It took
a long time to go from the inverse square law to the Poisson equation,
and from special relativity to GR. If both GR and MOND are correct (as
appears to be true empirically), then there must be a grander theory
encompassing both. But don't expect to derive it on the back of an
envelope in a few minutes.
Q: What about the Equivalence Principle?
A: The inertia interpretation of MOND breaks the symmetry of
the equivalence of gravitational
and inertial mass, which is one of the foundations of GR. This makes it
especially challenging to come up with a relativistic theory. Experimenters
have long searched for violations of the equivalence without success, so
it seems inviolable. However, these experiments are all performed in
acceleration fields eleven orders of magnitude greater than the acceleration
scale of MOND. Accelerations are not relative. Recall the Einstein
thought experiment of an observer in an elevator. The observer may not
be able to tell whether the weight that he feels is due to being stationary
on the surface of the Earth, or because he is being accelerated at 1 gee
out in space. But he knows very sell he is being accelerated!
You can do the Eotvos experiment here on Earth to arbitrary
accuracy and never discover MOND. Moreover, such experiments look for
composition-dependent differences: wood vs. gold, or some such. There is
nothing in MOND that is composition dependent.
Indeed, the galaxian data which support MOND are the only data
which test the Equivalence Principle in the relevant regime.
One fair interpretation is that it breaks
at small accelerations. We do not have a satisfactory
understanding of the origin of inertial mass. This
phenomenology may be a big clue.
Q: MOND can't actually be true, can it?
A: Empirically it works. No empirical piece of evidence
clearly contradicts* it. Its predictions come true where those of
dark matter based theories fail. Could be.
* - This was true when I wrote this web page in the late '90s.
Now (2013) I would say there are two pieces of apparently contradictory
evidence: the acoustic power spectrum of the cosmic microwave background (CMB),
and rich clusters of galaxies. Both of these are further discussed on
the main MOND page. In brief, the CMB isn't really
relevant [yet] as MOND provides no definitive prediction for it. It is
very well fit by the standard ΛCDM cosmology, but we've given ourselves
enough free parameters to guarantee a fit, so I'm not sure how impressed we
should be with that. Rich clusters show a residual mass discrepancy - one
still needs unseen mass, even with MOND! This is a clear problem for the
idea, though it is conceivable that some normal matter remains undetected
(we do have a missing baryon problem, after all). Even if this does indeed
falisify MOND, it does not alleviate the burden of explaining the observed
phenomenology: if MOND is wrong, why does it work as well as it does so often?
In Czech
In French
In Ukrainian
