People have always asked, and sought answers to, the big questions. How big is the Universe? How old? What is our place in it? And for as long as we could speak, we've been making up the answers.

We may be a privileged generation: the first in history to know the right answers to the big questions. According to Einstein's theory of General Relativity, the universe can not be static and eternal - it must either expand or contract. This was such a remarkable prediction that Einstein himself tried to deny it, inserting a fudge factor into his theory - the "cosmological constant" - to hold the universe steady. A decade later, Hubble discovered that the universe was in fact expanding. Einstein repudiated the cosmological constant as his "greatest blunder" and Big Bang cosmology was born.

Big Bang cosmology provides the modern framework for answering the big questions. But some basic questions remained rather open. How old is the universe? It depends on the expansion rate and the density of gravitating mass, which acts to slow the expansion. For many years, cosmology was described as the search for these two numbers. Measuring either to accuracy better than a factor of two has been a generations long struggle.

In the past decade, this long struggle has paid off. The Hubble Space Telescope measured the expansion rate to unprecedented accuracy. A host of experiments have constrained the mass density, culminating most recently with NASA's WMAP satellite which is widely credited for heralding in the era of "precision cosmology." Cosmic parameters are now constrained to typically 10% accuracy. We no longer say that the age of the universe is ten to twenty billion years. It is blithely stated to be 13.7 billion years. Just a decade ago, to quote a number past the decimal point would have drawn hoots of derision from one's cosmologist colleagues.

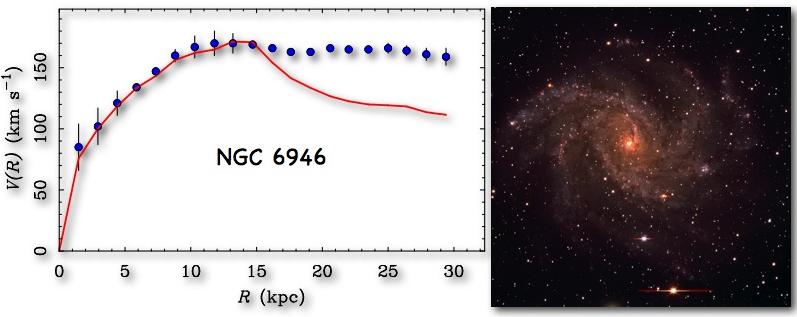
There is a dark side to this universe. The total amount of mass in the universe greatly exceeds that which we can see. Vera Rubin and her collaborators at the Department of Terrestrial Magnetism of the Carnegie Institution of Washington provided convincing proof for the existence of dark matter a quarter century ago with observations of the rotation curves of spiral galaxies. These remain flat when they should drop, if what we saw were all the mass present. That they don't requires dark matter - a result that eventually won her the National Medal of Science.

Modern measurements require that the mass of dark matter exceeds by a factor of 6 that believed to be in the form of ordinary matter. This dark matter must be in some novel form, widely believed to be some as yet undiscovered particle from the menagerie of particle physics. The existence of this dark matter is an absolute necessity to our modern cosmology, but has yet to be detected in the laboratory. If found, it would imply new physics beyond that of the stubbornly successful standard model of particle physics.

As if a universe in which most of the mass is invisible weren't strange enough, a variety of cosmic measures, most notably the brightness of supernovae seen to explode billions of light years distant, imply that the cosmic expansion rate is accelerating. This remarkable observation flies in the face of Einstein's pure gravity theory. Gravity between masses is strictly attractive; the cumulative density of mass in the universe, both luminous and dark, should act to slow the cosmic expansion. Instead, it seems to be speeding up!

Einstein himself provided the solution to this problem, in the form of his repudiated cosmological constant. Invented to hold the universe steady, such a fudge to his theory in effect plays the role of anti-gravity, pushing instead of pulling. This is precisely what we need to accelerate the expansion rate. Moreover, the fact that the push exceeds the pull means that there is even more "dark energy" (the modern generalization of Einstein's cosmological constant) than there is dark matter.

But wait. The anti-gravity that is dark energy means that General Relativity as Einstein originally formulated it - the version without a cosmological constant that successfully predicted the expansion of the universe - is not adequate to describe the universe. We have to modify it by adding dark energy. If we must generalize General Relativity, why stop there? Perhaps the need for dark matter is actually an indication of the need to modify gravity.

A few years after Rubin's pioneering observations, Moti Milgrom of the Weizmann Institute suggested as much. Indeed, many versions of modified gravity theories were tried and rejected. Usually, these supposed a change to the force law on some large scale size appropriate to galaxies. This approach does not work. The version suggested by Milgrom, MOND (for Modified Newtonian Dynamics), envisioned a modification of Newton's Second Law at a different kind of scale: at very low acceleration. (The centripetal acceleration experienced by a star orbiting about in a galaxy is roughly one part in 100,000,000,000 of that we feel on the surface of the earth.) Of all the modified gravity theories that had been suggested, this seemed theoretically to be the most outrageous. And yet, it worked like magic in fitting the rotation curves of spiral galaxies.

I have spent most of my career studying a class of objects known as Low Surface Brightness Galaxies. These diffuse galaxies rotate like spirals, but have a much larger mean separation between their stars. Like most astronomers, I had dismissed and largely ignored Milgrom's idea. When I learned he had made a number of very specific predictions for this class of objects, I assumed that my data would falsify his theory. Instead, I found it impossible to make sense of the data in terms of dark matter. In these ghostly galaxies where the dark matter must everywhere outweigh the luminous stars and gas, it was the ordinary matter that seemed to be calling the shots. To my growing incredulity, each observation that was puzzling in the context of dark matter turned out to be confirmation of one of Milgrom's long standing predictions.

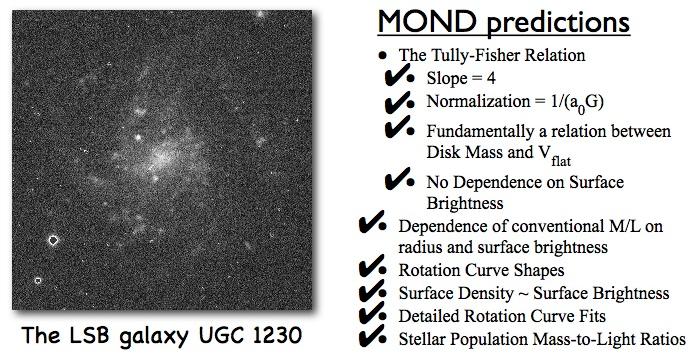
Still, Milgrom's idea seems to many too crazy to be possible. More crazy than dark matter. And dark energy. While it works uncannily well at describing the rotation curves of galaxies, it is less clear whether it works in other systems. Worse, it seemed utterly at odds with General Relativity.

This has changed recently with the advent of TeVeS. TeVeS was introduced in 2004 by Jacob Bekenstein, a physicist renowned for his work on the thermodynamics of black holes which set Steven Hawking on the path to discovering Hawking radiation. Bekenstein showed for the first time that it is indeed possible to write a theory encompassing both General Relativity and MOND. This is achieved by introducing scalar and vector fields in addition to the tensor field of General Relativity. The existence of such an all-encompassing theory removes the principle theoretical objection to modified gravity as a real physical alternative to dark matter.

It is early days for testing TeVeS. Can it provide a satisfactory description of cosmology? One test will be to see if it can explain the apparent need for dark energy as well as that for dark matter. If such a sweeping explanation is possible, it might well be preferable to the patchwork cosmology espoused at present. More locally, Bekenstein has suggested that TeVeS could be tested here in the solar system, as MOND-like effects might manifest themselves in small regions in interplanetary space where the gravitational effects of the sun and planets balance. There is a rich array of experiments to pursue which have the potential to lead to a true revolution in physics.

For the present, I remain perplexed by the stubborn success of MOND in individual galaxies. If the standard picture with dark matter is correct, this should not happen. So far, laboratory searches for dark matter have turned up empty. One begins to wonder - does the dark matter required by standard theory actually exist?

Throughout the history of the human endeavor to understand the cosmos, the limits on our observational horizon have flavored the answers to the big questions offered by our imaginations. We have made tremendous observational progress and genuinely know more now than ever previously. Have we finally found the right answers to the big questions? Or do these just open the door to still bigger questions?